Coverage Simulations for Band 2 Candidate Array (fpa143 at reduced bias)

I. Introduction

We present simulated depth-of-coverage maps using bad-pixel masks constructed from a WISE band-2 (4.7μm) candidate array. This is referred to as detector "FPA143_lowbias" in the test suite.

The simulations below are an extension of those for the same band-2 candidate (Coverage sims for fpa143), but with the array set at a reduced bias. The bias voltage was lowered from 250mV to 175mV. The purpose was to try and improve the bad-pixel performance by lowering the well depth (effectively from ~115,000e- to ~90,000e-). The main outcome is that the number of pixels that saturate in the A/D goes down from 0.3% to 0.02%.

Below we adopt the same assumptions, methodology and software as presented in previous simulation runs, e.g., Coverage sims for fpa141.

Inputs

The main input is a bad pixel mask image provided by the WISE Science Project Office. This was recieved as a 1024x1024 FITS image with bad pixels denoted with a value "1", and good pixels denoted with value "0".

For "FPA143_lowbias", bad pixels were identified using the same criteria as outlined in Coverage sims for fpa 141. For your informaion, ~2.9% of pixels in FPA143_lowbias (in the active region) are now declared as bad, compared to ~5.2% for the same array set at a higher bias voltage (see Coverage sims for fpa143).

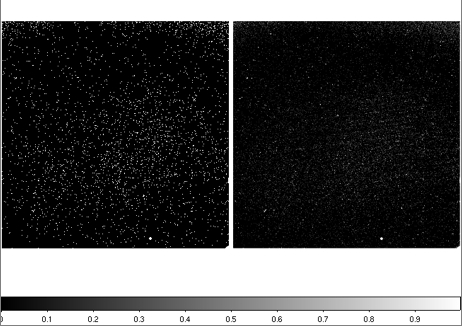Figure 1 - Left: bad-pixel mask from FPA143_lowbias provided by project office. Right: same mask after smoothing with an interpolation kernel (see below for details). Click on image to enlarge.

These masks can be downloaded in FITS format here:

bad_pix_tot143_175mV

bad_pix_tot143_smoothed_175mV

II. Results

We performed two simulations, each consisting of 100 (15-orbit) coverage realizations, corresponding to two bad-pixel masks: one using the default mask, and another using the same mask but smoothed with an interpolation kernel.

We present below coverage fractions, maps and histograms computed from the 100 realizations. These statistics represent the fraction of pixels (or area) within a simulated 2048x1024 central region with that depth-of-coverage. The means are computed over all realizations. The coverage maps represent those whose coverage fractions fall closest to the mean fractions.

1. Test Run #1 (Default Mask)

cov.  mean fraction (0 degrees)
4   0.00015978
5   0.00296004
6   0.03090069
7   0.15868088
8   0.29023703
9   0.27494447
10  0.15812363
11  0.06120418
12  0.01750559
13  0.00339246
14  0.00076413
15  0.00022826
16  0.00079893
17  0.00009986

cov.  mean fraction (90 degrees)
4   0.00017680
5   0.00314759
6   0.03228805
7   0.16117956
8   0.28939181
9   0.27327625
10  0.15739666
11  0.06147846
12  0.01688073
13  0.00328725
14  0.00069765
15  0.00029967
16  0.00039956
17  0.00009989

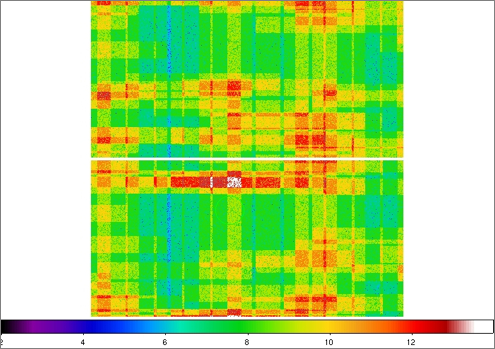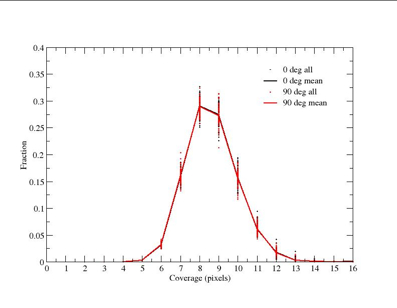Figure 2 - Coverage maps for Test 1 (default mask): top = 0 degrees; bottom = 90 degrees.	Figure 3 - Coverage distributions for Test 1 (default mask).
Left: false color JPEG images of coverage maps for the default mask. The color bar at the bottom corresponds to the approximate coverage depth. Right: corresponding coverage distribution with fractions normalized to unity. Dots represent the 100 individual realizations. The lines go through the mean fractions from all realizations. Click on thumbnails to see full-size JPEG maps.

Summary

• For the default FPA143_lowbias bad-pixel mask that uses all the "bad" criteria, ~48% of an area on the ecliptic equator (the mean at 0 or 90 degrees) will have fewer than 8 coverages. <0.1% will have fewer than 4 coverages.
• As a reminder, a minimum coverage of 4 over at least 95% of the whole sky is the mission requirement. But note, our simulation area effectively represents ~0.7% of the sky centered on the ecliptic equator when compared to the full ecliptic latitude range [-90 to 90 degrees].
• The mode coverage is ~8. The RMS scatter about the mean fraction at this value is ~ +/-2.5% (both 0 and 90 degrees) and is representative at other coverages;
• For 100 realizations, the standard error in the mean fractions quoted above is of order RMS/sqrt(100) ~ +/-0.25%;

2. Test Run #2 (with Kernal Smoothing)

cov.  mean fraction (0 degrees)
5   0.00031282
6   0.01524354
7   0.14330952
8   0.29332132
9   0.29246380
10  0.16833764
11  0.06520483
12  0.01742533
13  0.00331735
14  0.00073898
15  0.00027484
16  0.00004997

cov.  mean fraction (90 degrees)
5   0.00033576
6   0.01560488
7   0.14146726
8   0.29271856
9   0.29064004
10  0.17138096
11  0.06486780
12  0.01805614
13  0.00365839
14  0.00088919
15  0.00028104
16  0.00009992

Figure 4 - Coverage maps for Test 2: top = 0 degrees; bottom = 90 degrees.	Figure 5 - Coverage distributions for Test 2.
Left: false color JPEG images of coverage maps for Test 2 (including smoothing from an interpolation kernel). The color bar at the bottom corresponds to the approximate coverage depth. Right: corresponding coverage distribution with fractions normalized to unity. Dots represent the 100 individual realizations. The lines go through the mean fractions from all realizations. Click on thumbnails to see full-size JPEG maps.

Summary

• For the same FPA143_lowbias bad-pixel mask convolved with a model interpolation kernel, ~45% of an area on the ecliptic equator (the mean at 0 or 90 degrees) will also have fewer than 8 coverages. There are no areas with fewer than 4 coverages;
• The mode coverage is ~8.5. The RMS scatter about the mean fraction at this value is ~ +/-3% (both 0 and 90 degrees) and approximately representative at other coverages;
• For 100 realizations, the standard error in the mean fractions quoted above is of order RMS/sqrt(100) ~ +/-0.3%;

III. Conclusions

• Given the default FPA143_lowbias (band-2) bad-pixel mask, <0.1% of an area on the ecliptic equator will have fewer than 4 coverages, and ~48% will have fewer than 8 coverages (see Figure 3). The simulated area effectively represents ~0.7% of the sky.
• With inclusion of smoothing from an interpolation kernel as represented by a model PRF fit, there are no areas with coverage depths <5, while ~45% will have fewer than 8 coverages (see Figure 5). For your information, interpolation kernels will be used for optimal Atlas image generation.
• The reason for these slight (but significant) drops in areas with low-coverage when kernel smoothing is present is due to a "smearing" of good pixel information into adjacent bad pixels (mainly because there are more good pixels than bad ones). This effectively increases the depth-of-coverage at the locations of bad pixels on the sky.
• The impact of an interpolation kernel on increasing the effective depth-of-coverage at bad pixels is maximized if the bad pixels are more-or-less randomly distributed as single isolated pixels. If the same number of bad pixels were in larger concentrations, a large fraction would be shielded by the PRF tails of good pixels and thus, the average depth-of-coverage is expected to decrease.
• Note: the above does not mean that bad pixels can be completely mitigated by the smearing effect of the PRF. There is still a net loss of information when bad pixels are present in that the effective coverage is slightly lowered at the location of good pixels that are next to bad ones. Also, smearing by the PRF itself results in reduced point source sensitivity by an amount proportional to the square root of the equivalent number of "noise pixels" contributing to a flux measurement. The number of noise pixels is characteristic of the PRF. For more details on kernel smoothing and noise pixels, see: Use of Smoothing Kernals.